Volume is the quantification of the three-dimensional space a substance occupies. The SI unit for volume is the cubic meter, or m3. By convention, the volume of a container is typically its capacity, and how much fluid it is able to hold, rather than the amount of space that the actual container displaces.
Volumes of many shapes can be calculated by using well-defined formulas. In some cases, more complicated shapes can be broken down into simpler aggregate shapes, and the sum of their volumes is used to determine total volume. The volumes of other even more complicated shapes can be calculated using integral calculus if a formula exists for the shape's boundary. Beyond this, shapes that cannot be described by known equations can be estimated using mathematical methods, such as the finite element method. Alternatively, if the density of a substance is known, and is uniform, the volume can be calculated using its weight. This calculator computes volumes for some of the most common simple shapes.
The volume of sphere is the capacity it has. The volume of sphere is measured in cubic units, such as m3, cm3, in3, etc. The shape of the sphere is round and three-dimensional. It has three axes as x-axis, y-axis and z-axis which defines its shape.
All the things like football and basketball are examples of the sphere which have volume. A simple check on any formula for area or volume is a dimensional check. Area is the two-dimensional amount of space that an object occupies. Area is measured along the surface of an object and has dimensions of length squared; for example, square feet of material, or square centimeters. Volume is the three-dimensional amount of space that an object occupies.
Volume has dimensions of length cubed; for example, cubic feet of material, or cubic centimeters (cc's). Any object displaying the above mentioned characteristic is said to have a spherical shape. If the inside of the sphere is empty, it is referred to as a spherical shell or a hollow sphere. If the inside of the sphere is filled, it is called as a solid sphere . One can calculate theweightof any object by multiplying thedensityof the material by the volume of the object.
On this slide, we list some equations for computing the volume of objects which often occur in aerospace. There are similar equations for computing theareaof objects. The magnitude of theaerodynamic forcesdepends on the surface area of an object, while thegravitational forceand certainthermodynamic effectsdepend on the volume of the object. The equations to compute area and volume are used every day by design engineers. Calculating volume and surface area of sphere play an important role in mathematics and real life as well.
Formulas for volume & surface area of sphere can be used to explore many other formulas and mathematical equations. A sphere is the shape of a basketball, like a three-dimensional circle. Just like a circle, the size of a sphere is determined by its radius, which is the distance from the center of the sphere to any point on its surface. The formulas for the volume and surface area of a sphere are given below.
If you ever wondered what's the volume of the Earth, soccer ball or a helium balloon, our sphere volume calculator is here for you. It can help to calculate the volume of the sphere, given the radius or the circumference. Also, thanks to this calculator you can determine the spherical cap volume or hemisphere volume. In this example you need to calculate the volume of a very long, thin cylinder, that forms the inside of the pipe. The area of one end can be calculated using the formula for the area of a circle πr2. The diameter is 2cm, so the radius is 1cm.
The area is therefore π × 12, which is 3.14cm2. This online calculator will calculate the 3 unknown values of a sphere given any 1 known variable including radius r, surface area A, volume V and circumference C. It will also give the answers for volume, surface area and circumference in terms of PI π. A sphere is a set of points in three dimensional space that are located at an equal distance r from a given point . A sphere is a round shape solid object in three-dimensional space. It can be defined as the set of points that are all at the same distance from a given point .
The perfect example of the sphere is the globe and ball. The following figure shows a sphere shape whose radius is r, and the diameter is d. A glass dome for a lighting fixture is in the shape of a hemisphere. The circumference of the great circle of the hemisphere is 12π inches.
Which statements about the hemisphere are true? The total surface area is 108π square inches. The total surface area is 144π square inches. The total surface area is 432π square inches. The total surface area is 36π square inches. This distance r is the radius of the sphere, and the given point is the center of the sphere.
A sphere with radius R is a three-dimensional geometrical object where the distance between the center and any point on the surface equal to R. Every plane section of a sphere is a circle. A plane section through the center results in a largest possible circle with radius R. For any other value for the length of the radius of a sphere, just supply a positive real number and click on the GENERATE WORK button.
They can use these methods in order to determine the surface area and volume of parts of a sphere. A sphere is a three-dimensional solid with no base, no edge, no face and no vertex. Sphere is a round body with all points on its surface equidistant from the center. The volume of a sphere is measured in cubic units. A sphere is a three-dimensional solid with no face, no edge, no base and no vertex. It is a round body with all points on its surface equidistant from the center.
The volume of a sphere is determined by the three coordinates x, y, and z. Because a three-dimensional object will lie on all three axes. Volume is measured in cubic meters, cubic feet, cubic inches, and similar units. It is represented by symbols cm3, m3, in3, and so on.
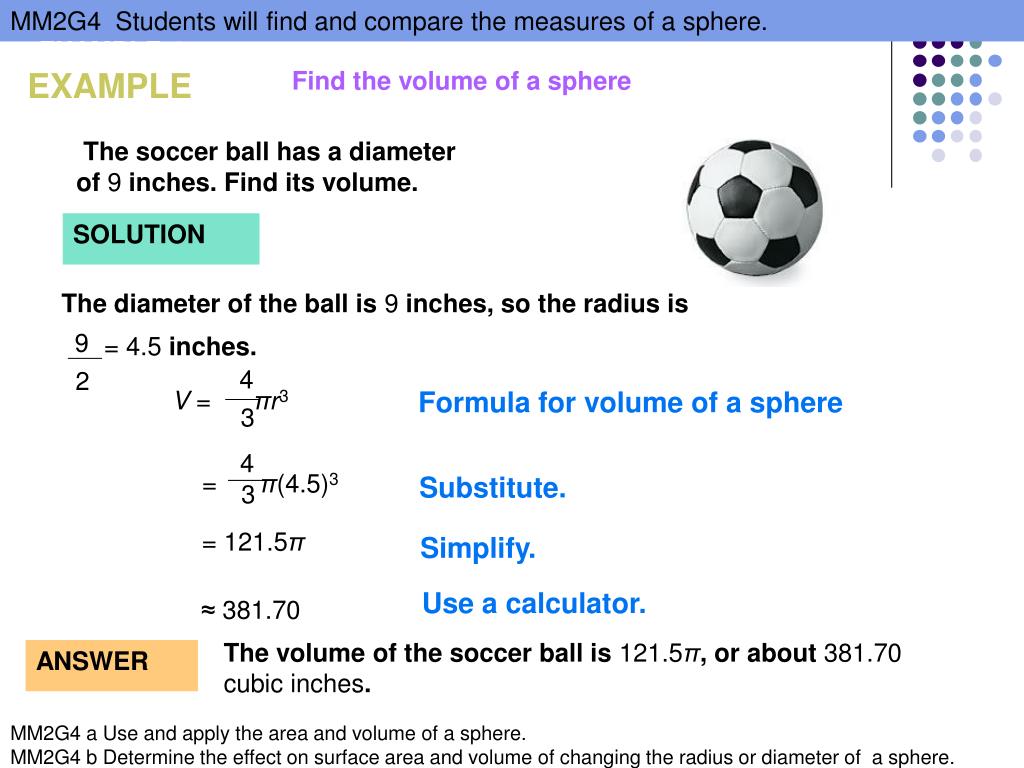
If the baseball has a surface area of 9π, then I can set that equal to the formula 4πr2 and solve for r. The radius is 3/2 inches, so I double that to find the diameter. The diameter of the ball is 3 inches, which is greater than the allowed range of diameters. The volume of a sphere is the amount of space occupied by it. For a hollow sphere like a football, the volume can be viewed as the number of cubic units required to fill up the sphere.
Given radius of sphere, calculate the volume and surface area of sphere. In this calculation you can calculate the volume of a sphere with a number of given input values, such as radius, diameter, circumference. You also have a number of different input units and can choose output unit according to your likings. A set of points in a space equally distanced from a given point $O$ is a sphere. The point $O$ is called the center of the sphere. The distance from the center of a sphere to any point on the sphere is called the radius of this sphere.
A radius of a sphere must be a positive real number. The segment connecting two points on the sphere and passing through the center is called a diameter of the sphere. All radii of the sphere are congruent to each other.
A sphere can be obtained by rotating a semicircle about the diameter. Two spheres of the same radius are congruent. The pi in the formula is the constant that we use when finding the circumference of a circle, and the radius, as you might remember, is half the length of the diameter. The last thing is that the radius is cubed. This relates to the fact that in the end we are solving for volume, which has three dimensions.
So far we've calculated the volume of cubes, rectangular tanks, and cylinders. Using that information, how do you think we would calculate the volume of a sphere? What variables do you think you would use? Take a few moments to think about it before we go through the explanation below. The following video shows how to solve problems involving the formulas for the surface area and volume of spheres.
A globe of Earth is in the shape of a sphere with radius 14[/latex] centimeters. Round the answer to the nearest hundredth. There are so many examples of spherical objects in our day-to-day life. Just remember or derive the formula and calculate the volume for applications. Volume is a fixed quantity and can be found using Archimedes' principle. According to Archimedes, if the solid sphere is dropped in the container filled with water, the volume of water displaced will be equal to the volume of the sphere.
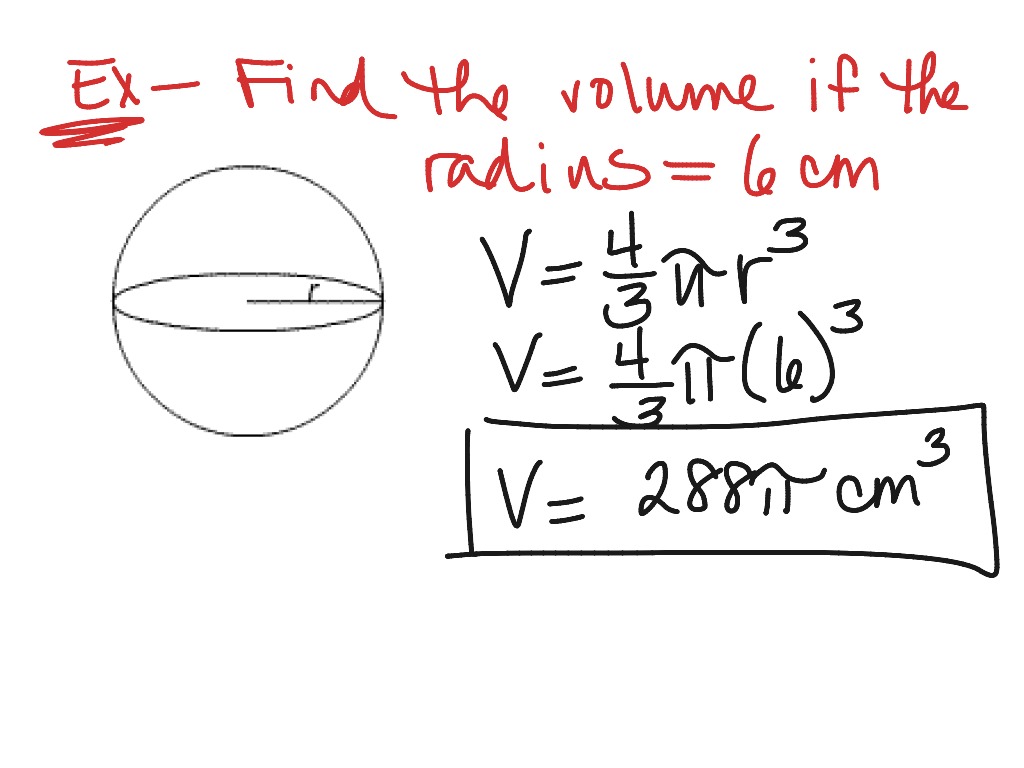
The volume will change when the values of the diameter or radius of the sphere will change. Otherwise, the formula for the volume of the sphere will remain the same. The formula for the volume of a sphere is 4/3 times pi times the radius cubed.
Cubing a number means multiplying it by itself three times, in this case, the radius times the radius times the radius. A regulation baseball must have a diameter between 2.87 and 2.94 inches. The surface area of a particular baseball is 9π square inches. Is the baseball within the range of regulation size?
The sphere is a fundamental object in many fields of mathematics. Spheres and nearly-spherical shapes also appear in nature and industry. Bubbles such as soap bubbles take a spherical shape in equilibrium.
The Earth is often approximated as a sphere in geography, and the celestial sphere is an important concept in astronomy. Manufactured items including pressure vessels and most curved mirrors and lenses are based on spheres. Spheres roll smoothly in any direction, so most balls used in sports and toys are spherical, as are ball bearings. Let us see some examples of calculating the volume of spheres of different dimensions. The volume of a 3 -dimensional solid is the amount of space it occupies.
Be sure that all of the measurements are in the same unit before computing the volume. The diameter of a sphere is the longest line that is inside the sphere and that passes through the center of the sphere. A sphere is a three-dimensional shape that is perfectly symmetrical and round in shape.
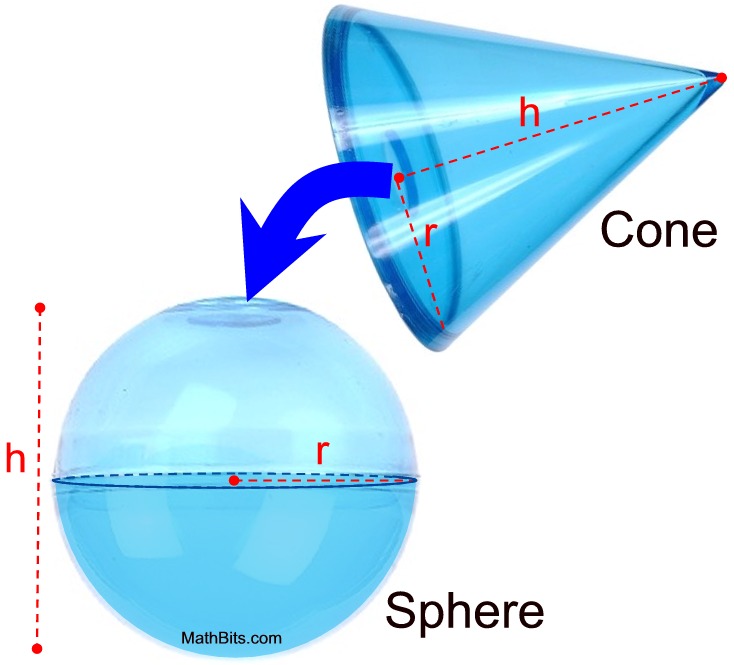
Some examples of spheres are a ball, a globe, etc. Consider a sphere and two right circular cones of same base radius and height such that twice the radius of the sphere is equal to the height of the cones. The volume of a sphere is measured in cubic units, i.e., m3, cm3, in3, ft3, etc. Just split the solid up into smaller parts until you reach only polyhedrons that you can work with easily. This concept can be of significance in geometry, to find the volume & surface area of sphere and its parts.
Real life problems on volume & surface area of sphere are very common, so this concept can be of great importance of solving problems. The problem has to be solved in two simple steps. First we have to find the empty volume in the beginning, and then find the time it takes to fill that volume. Therefore, we have to calculate the volume of a semi-sphere, which is also the volume filled with water. The geometry of the sphere was studied by the Greeks. The volume and area formulas were first determined in Archimedes's On the Sphere and Cylinder by the method of exhaustion.
Zenodorus was the first to state that, for a given surface area, the sphere is the solid of maximum volume. In this article, we will learn the details of the volume of a sphere, its definition, formula or equation calculation, unit in Gallons or liters, along with so many examples. Assume that the volume of the sphere is made up of numerous thin circular disks which are arranged one over the other as shown in the figure given above. The circular disks have continuously varying diameters which are placed with the centres collinearly. A thin disk has radius "r" and the thickness "dy" which is located at a distance of y from the x-axis. Thus, the volume can be written as the product of the area of the circle and its thickness dy.
Archimedes' principle helps us find the volume of a spherical object. It states that when a solid object is engaged in a container filled with water, the volume of the solid object can be obtained. Because the volume of water that flows from the container is equal to the volume of the spherical object.
Calculate the volume of all common geometrical shapes, such as cubes, spheres, pyramids, cones etc. Calculator for Volume and Diameter of a Solid SphereThis calculator calculates for the volume and diameter of a solid sphere. Enter any one value and leave the value to be calculated blank. The number of decimal places in the calculated value can also be specified. A spherical solid metal of a radius of $16$ inches is melted down into a cube. Use $\pi \approx 3.14$ and estimate your answer to the nearest whole number.